This article is cited in
1 paper
Applied mathematics
On algorithm of numbering the spanning trees in a connected graph
V. V. Popov Volgograd State University
Abstract:
Let
$G = (V, E)$ be a finite connected graph without multiple edges or loops.
We consider the task about the numbering of all the spanning trees of
$G$.
In [2, p. 180, p. 191] described a method of solution of this task,
based on the properties of minors of an incidence matrix of
$G$.
In the same place (p. 191–193) gave algorithm of four Japanese mathematicians
(Kasahara Y., Tezuka K., Ling Shun Tong, Kitahashi T., [6]),
reduced the task to removal of brackets in the product of formal sums of edges of
$G$.
Here we concider the method based on lexicographical order on the set of
all sequences of edges of
$G$.
We will remind that a spanning tree
$T$ of the graph
$G$ is a tree consisting
of edges of
$G$ and connecting all the vertices of
$G$.
We shall assume that
$V =\{ 1,2, \ldots, n\} $, where
$n$ is a
number of vertices of
$G$. If
$a, b \in V$,
then
$ (a, b) $ will be designated the edge with end-points
$a$ and
$b$.
Let
$T$ be a spanning in
$G$.
Then the set of his edges can be written in the form
\begin{equation}
(a_1, b_1), (a_2, b_2), \ldots, (a_ {n-1}, b_ {n-1}), \tag{A}
\end{equation}
where
\begin{equation}
a_i<a_{i+1} \ {\rm or} \ a_i=a_{i+1}\ \text{and} \ b_i<b_{i+1}, \ \text{where}\ i=1,2,\ldots, n-2.
\tag{B}
\end{equation}
On a set of sequences of in the form of (A) with the additional condition (B)
we will consider a lexicographic order.
The smallest (with respect to this order)
spanning tree will be the tree
$T_0$ with edges
$ (1,2)$,
$(1,3)$,
$ (1,4)$,
$\ldots$,
$ (1, n) $
provided
$(1,b) \in E$
for
$b=2,3, \ldots, n$.
The greatest spanning tree
$T_1$ will be
$ (1, n) $,
$ (2, n) $,
$ (3, n) $,
$\ldots$,
$ (n-1, n) $
under a similar condition.
Touching all sequences of a look (A) in ascending order
between
$T_0$ and
$T_1$
and checking lack of cycles at the corresponding
sets of edges,
we will be able to get a list of all spanning trees.
We will give results of work of the appropriate computer program.
Example 1. For complite graph
$K_n$ on
$n$ vertices
for
$n\leq 9$ the
lists of all the spanning trees are obtained.
Due to Kely theorem
$K_n$ has
$n^{n-2}$ spanning trees.
For
$n=9$ thos number is equal
$4\,782\,969$.
Example 2. Let
$G$ be a graph on
$12$ vertices in figure 1 (in the left).
Then
$G$ has
$2\,415$ spanning trees.
Let
$P$ be a finite set of points in the plane
$R^2$.
Then
$G = (V, E) $ is a plane geometrical graph on top of
$P$,
if
$V\subset R^2$ and egdes of
$G$ are stright-line segments with the
end-points in
$P$ and two edges of
$G$ intersects only in points of
$P$ [3].
If
$G$ is a tree and
$V=P$, then
$G$ called a spanning tree on top of
$P$.
Example 3. Let
$P$ be a set of square tops,
and also its center. Then there are
$45$ spanning trees on top of
$P$.
Example 4. Let
$P$ consists of square tops, and also
middle of its parties. Then there are
$3\ 273$ spanning trees on top of
$P$.
Example 7. We will consider a set
$P=L_{n,m}$
consisting of
$n\cdot m$ points on the plane:
$$L_ { n, m } = \{ (i, j): i=1,2, \ldots, n, j=1,2, \ldots, m\}, $$
where
$n, m\geq of 1$ are integers.
Thus,
$L_ { n, m } $ is a uniform lattice which points lie on
$n$
horizontal straight lines and for
$m$
vertical straight lines.
The numbers
$St(P)$ of a spanning trees on top of this lattice
for small
$n$ and
$m$ it is specified in the following table:
At transfer the spanning trees it is possible to carry out a filtration.
For example, it is possible to keep only those trees in the final list,
which degrees of vertices not exceeded some number
$r$.
So, the complete graph on
$8$ vertices has
$8 ^6=262\,144$
spanning trees. Among them
$201\ 600$ spanning trees
which degree of vertices don't exceed
$3 $,
and
$20\,160$ spanning trees with degree of vertices
$\leq 2$.
Some modification of the main algorihm
allows to receive the list of all crossing-free geometrical graph
on top of
$P$ [3].
For example, if
$P$ consists of square tops, and also
middle of its parties, the number of such a graph on top of
$P$
is equal to
$21\,795$ (this number included also the empty graph).
If to add to
$P$
the center of a square, this number will become to
$167\,570$.
In work is considered
also a task about creation of all triangulations of a finite set
$P\subset R^2$.
The algorithm of the solution of this task is available in [3].
The alternative description of algorithm of search is provided in this work.
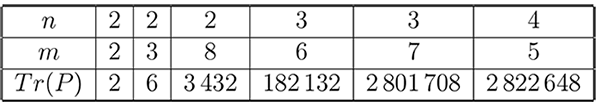
Example 9. Let
$P=L_{ n, m } $ be a uniform lattice on the plane
(see example 7). Then the number
$Tr(P)$ of triangulations of
$L_{ n, m } $
for small
$n$ and
$m$ is specified in the following table:
Keywords:
connected graph, planar graph, spanning tree, the number of spanning trees, triangulation, the number of triangulations.
UDC:
517.518.85, 517.27
BBK:
22.144
DOI:
10.15688/jvolsu1.2015.2.1